Author: admin-agc

Introduction

The advent of low-cost antenna analyzers and vector network analyzers (VNAs) has resulted in a renewed interest in an 80-year old tool called the Smith chart, a graphical device used to view the characteristics of RF transmission lines, antennas, and matching circuits, and to aid in the design of those systems.  But although questions regarding the chart appear in the Amateur Extra License exam, the current Question Pool has a mere 11 questions on the topic, leaving a full appreciation of the tool to the more inquisitive student. Here then, is a brief introduction to the Smith chart.

Motivation

Why use a seemingly-archaic tool such as the Smith chart, when calculators and software are readily available?  For one, the chart remains useful as a visualization tool, representing a wealth of information in a very precise and intuitive manner.  In addition, the knowledge gained in its use adds to our general knowledge of radio systems. And that’s what ham radio’s all about, right? Otherwise, we may as well use a telephone or the Internet to communicate over large distances!  And don’t worry; we’ll keep the equations to a minimum (or in footnotes).

Phillip Smith, 1ANB (SK), was an electrical engineer who graduated from Tufts College in 1928, and then went to work in the radio research department at Bell Telephone Laboratories.  Fascinated by the repetitive nature of the impedance variation along a transmission line and its relation to the standing-wave amplitude ratio and wave position, Smith devised an impedance chart in which standing-wave ratios were represented by circles. In 1939, he had an article published in Electronics Magazine describing what later came to be known as the Smith chart.

Derivation

The Smith chart provides a concise way to view a number of different characteristics of RF systems, such as impedance and voltage standing-wave ratio (VSWR). We’ll use an example to show how the Smith chart was developed.

Radio operators are inherently familiar with the concept of standing-wave ratio, i.e., a measure of how well a load is matched to a transmission line. A slightly more obscure concept is that of the reflection coefficient. Notated using the Greek letter gamma (Γ), the reflection coefficient describes how much of an electromagnetic wave is reflected by an impedance discontinuity such as a mismatched load. (For a perfect termination, Γ=0, an open termination yields G=1, and a short results in Γ=-1.) Being a complex quantity, Γ can convey both the magnitude and phase of the reflected wave, at a particular frequency.^{[1], [2]}

We can measure Γ by connecting a VNA to a device under test (DUT), “sweep” a band of interest, and download the data.  Our DUT for this example is a 50-ohm coaxial transmission line terminated by a 40m OCF dipole.

First, let’s plot the real and imaginary components of Γ for this DUT on a rectilinear graph. See Figure 1: the green marker is at 14.175 MHz.

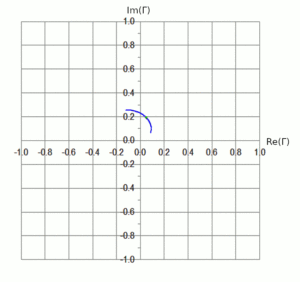
Reading off the chart, we see the following values:

frequency	14.000 MHz	14.175 MHz	14.350 MHz
Γ	-0.12 + j 0.26	0.05 + j 0.20	0.08 + j 0.07

Because complex numbers can also be represented in polar form, we can also plot Γ on a polar plot, as in Figure 2. On this plot, the same green marker is now at a magnitude (denoted as |Γ|) of about 0.2 (i.e., its distance from the center or origin), and a phase angle of about 75°. (Note that the curve did not change shape, only the axes of the graph did.) It’s useful to note that the magnitude of the reflection coefficient can never exceed 1.0, as this would imply that power is somehow “created” by the load. This means that Γ is constrained to be within this circular space, such that |Γ|≤ 1.

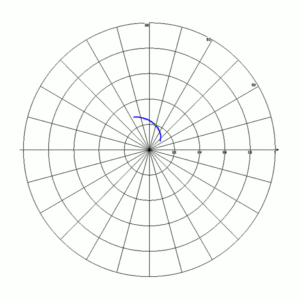
Smith was no doubt very familiar with the polar type of data presentation, for a practical reason. Back in the day, it would have been rather difficult to measure Γ directly as a complex quantity, due to the limitations of test equipment. But it would have been much easier to measure the polar representation: what was required was an oscilloscope and directional couplers.  With these, one could measure the magnitude and phase angle of a wave reflected from a termination, and plot them on a polar graph of the complex reflection coefficient. A simple trigonometric transformation converts these to the rectangular format.^[1]

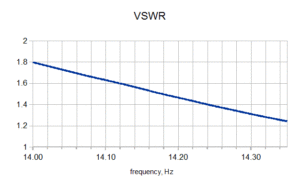
There are several quantities that can be derived from the reflection coefficient. For one, the VSWR is easily calculated from the magnitude of Γ, and is shown in Figure 3.^[2]

Another is the complex impedance, which is also easily calculated from the reflection coefficient at that same “reference plane,” i.e., the point at which the measurement is taken. Because this transformation is so elegant (and important), we present it here:

\displaystyle Z_{L}=\frac{1+\Gamma}{1-\Gamma}\cdot Z_{0}

Equation 1

---

^[1] Because RF components behave differently at different frequencies, engineers use complex numbers to describe impedances and related concepts. The impedance measured at the input to an antenna, at a particular frequency, is expressed as a complex number in the form Z = R + jX, where R represents the real value of the impedance (in ohms), X represents the imaginary value (also in ohms), and j is the imaginary constant \sqrt{-1}.

^[2] The quantity Γ is also denoted by the scattering parameter s₁₁. S-parameters generally describe the input-output relationship of RF networks; another such s-parameter is s₂₁, which represents the power transferred to a “Port 2” from a “Port 1” of a network.

---

In other words, knowing the reflection coefficient Γ and the characteristic impedance Z₀ of a transmission line connected to a load, we can directly calculate the load impedance Z_L.^[3]  (As we know, in radio engineering, Z₀ is usually 50Ω; video plants commonly use 75Ω, and telephone networks use 600Ω.)

Applying this transformation to our example data, we can produce a graph of the complex load impedance, as in Figure 4, with some interesting observations.  First, although the curve looks similar to that of the reflection coefficient, the shape cannot be the same for the following reason: as we learned before, while the reflection coefficient is bounded to a magnitude of 1, the impedance can take on values from zero to infinity. Indeed, if Γ is close to 1, the load impedance can be very high, usually on the order of thousands of ohms.

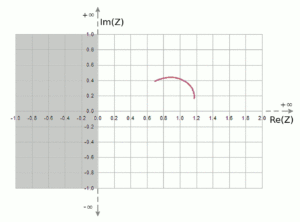
The other useful observation is that, while Γ can have both positive and negative real and imaginary components, the real (resistive) part of the complex impedance can only be positive,^[4] and can range from 0 to +∞, while the imaginary part can range anywhere from −∞ to +∞.

From a practical standpoint, this means that conventional (linear) representations of both reflection coefficient and impedance suffer from a number of shortcomings: impedance plots that cover a large extent (e.g., traversing a region close to Γ = 1) would be unwieldy and lack precision; simultaneous plots of different systems would be hard (or impossible) to compare; multiple graphs would be needed to convey information that is intrinsically related; and the conventional representations would be limited to some applications of analysis, while impeding the practicality of system design.

---

^[1] If we denote the phase of Γ by θ, then \Gamma=\left |\Gamma \right |\cdot (\cos \theta +j\cdot \sin \theta) .

^[2] VSWR=\displaystyle \frac{1+\left |\Gamma \right |}{1-\left |\Gamma \right |} .

^[3] Keep in mind that Z_L and Γ are complex quantities, so complex arithmetic must be used!

^[4] There is no such thing as negative resistance (!), except in tunnel diodes, but that’s another story.

---

The Smith chart

Phillip Smith understood these limitations, and set forth to overcome them.  He brilliantly realized that the various concepts of impedance, reflection, and VSWR could become graphically interrelated by means of the right coordinate transformation – and this would solve the “infinite extent” problem, as well.

Smith’s insight was to start with a rectangular grid of complex impedance, and then warp the axes according to the transformation given in Equation 1.  This has the effect of throwing away the negative real (resistance) part, and wrapping the imaginary (reactance) axis so that the points at −∞ and +∞ meet each other. Thus was born his eponymous chart, shown in Figure 5.

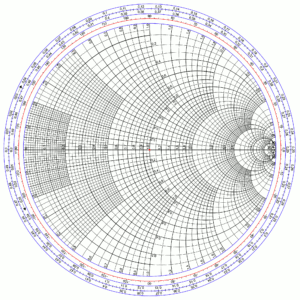
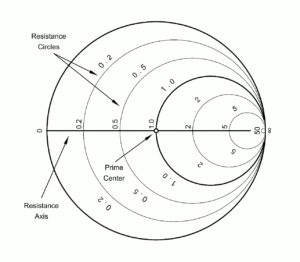
The chart consists of a number of internally-tangent circles, and arcs of circles; the number of circles and arcs is simply a function of the desired precision, and charts can also be constructed that “zoom-in” on a particular region of interest. Looking first at the circles, we see they are all internally tangent at a point on the right-hand-side, as seen in Figure 6.  Each of these circles represents a constant real (resistive) component, with the circles ranging from 0 ohms (as indicated at the extreme left of the horizontal line – the real, or resistance, axis) to +∞ ohms (as indicated at the extreme right of the horizontal line).

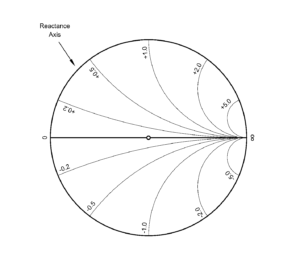
Intersecting these circles are arcs of circles that have centers lying on a line (not shown) that is perpendicular to the horizontal line, and which all pass through the point at +∞ ohms. (See Figure 7.) Each of these arcs represents a constant imaginary (reactive) component.  The outermost full circle is the imaginary, or reactance, axis.

A few definitions and we’re there. You’ll recall from Equation 1 that the load impedance Z_L can be calculated by knowing Γ and the characteristic impedance Z₀.  We can rewrite this equation in a form that normalizes the impedances by dividing both sides of the equation by Z₀, which produces Equation 2:

\displaystyle \frac{Z_{L}}{Z_{0}}=\frac{1+\Gamma}{1-\Gamma}

Equation 2

With this normalization, we can use the Smith chart for any characteristic impedance, as long as we remember that the point at the center represents that characteristic impedance. Inspecting the chart, and from the above definitions, we see that the point at the chart center has a value of 1 + j·0, i.e., 1.

The last component to observe is the outermost scale, which is calibrated in degrees and fractions of a wavelength.  Among the useful properties of the Smith chart is the fact that adding a length of transmission line has the effect of rotating the complex reflection coefficient clockwise around the chart center, by an angular amount that is proportional to the added electrical length divided by the operating wavelength.

For example, if we add 4 meters of RG-8X transmission line (with velocity factor 0.82) to a system operating at a wavelength of 21.2 meters, the curve will rotate^[1] by the following angle:

\displaystyle \varphi =\frac{1}{0.82}\cdot \frac{4}{21.2}\cdot 720 \degree = 166 \degree

---

^[1] One wavelength corresponds to two complete rotations around the Smith Chart, hence the 720° factor.  Note that adding multiples of ½ wavelength simply brings us back to the same point!

---

Returning to our DUT, we can now appreciate the utility of this marvelous tool.  Using the data from Figure 1, if we now take the reflection coefficient curve of our system, and “drop” it onto the Smith chart (see Figure 8), we can read off the following normalized impedance information (and calculate the actual impedance, shown on the table’s third line, by multiplying by Z₀ = 50Ω): ^{[1], [2]}

 

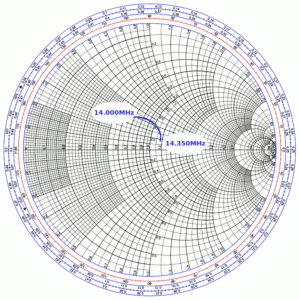
	14.000 MHz	14.175 MHz	14.350 MHz
ZL/Z0	0.68 + j 0.38	1.02 + j 0.41	1.16 + j 0.15
phase(Γ)	116°	76°	38°
ZL , Ω	34 + j 19	51 + j 20.5	58 + j 7.5

---

[1] It’s important to realize that the scales on the chart are actually performing the calculation for us; we started with a curve of Γ on a conventional polar graph, and copied that same curve onto the Smith chart, where it now represents Z. It’s only the axes that have changed.

[2] To get the phase of a point, draw line from the center through the point, and extend it to the outer scales, where you can read it off the 0-180° scale.

---

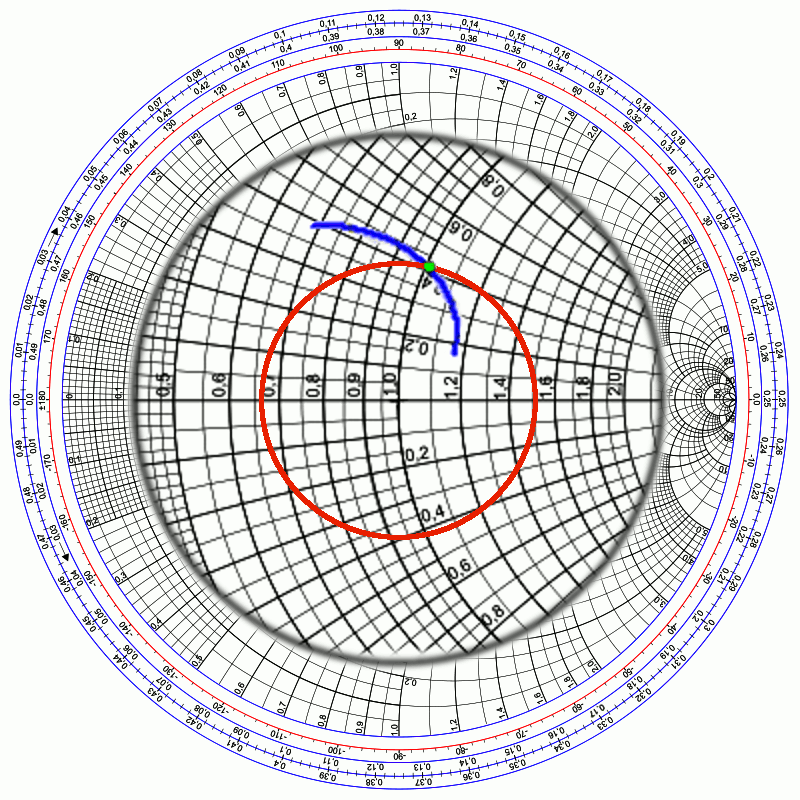
You may have noticed that the VSWR formula given previously looks curiously similar to the normalized impedance formula given at Equation 2, which leads to another wonderful property of the Smith chart: if you take any point on an impedance plot, and rotate it, around the center, to land on the right-hand half of the resistance axis, the value at that axis is the VSWR at that frequency! (See magnified center at Figure 9.) Note how rotating the green dot would intersect the horizontal axis at the value 1.5, which is what we calculated earlier. In fact, any point on the red circle has a VSWR of that same value. So, when analyzing (or designing) a system with a target VSWR, make sure that the normalized impedance values over the entire frequency of interest remain inside such a circle.

We specifically chose a limited range of frequencies to keep the visuals simple; in practice, extending the frequency over a wider range leads to some very interesting results! Note too, that while many of these measurements were originally done using paper-and-pencil (and drafting tools), we can easily do the equivalent by using graphical software that directly interfaces to a modern VNA.

Further Reading

Unfortunately, Smith’s own treatise, Electronic Applications of the Smith Chart in Waveguide Circuit and Component Analysis, published in hardcover in 1969, has been out of print for several years, and used copies fetch a premium price. Readers interested in an in-depth elaboration of the chart, as well as examples of using the chart to design transmission elements like matching networks, are directed to the ARRL Handbook Supplemental Articles and The ARRL Antenna Book.  Many online resources are available as well, including an Oral History of Phillip Smith, and the Wikipedia page, Smith Chart.

---

Afterword

As of this writing, the Smith Chart on the Wikipedia page has a small typo; can you find it?

The author has an original copy of Smith’s book, complete with intact transparent overlays — one of his cherished antiques.

–agc